In statistics and research, students often find themselves confronted with the objective of conducting a study. While there are many methodologies to do so, one especially promising is the hypothesis testing. There, you can formulate two hypotheses that exclude each other and use your findings of the study to determine which one is correct. The following article will teach you what hypothesis testing is and how you use it in your academic writing.
Hypothesis testing in a nutshell
In hypothesis testing, sample data is used to determine whether a hypothesis is plausible.
Definition: Hypothesis testing
Hypothesis testing is a statistical method where an analyst or researcher attempts to study a statement related to a given population parameter. The reason for this analysis and the data currently available determines the research methodology that the analyst will apply. The hypothesis test itself determines whether a hypothesis can be accepted as most plausibly true or not.
Step-by-step guide
Conducting a statistical hypothesis testing may look a lot more complicated than it actually is. The following steps will lead you to a successful result.
Costruct the hypotheses
The first step is to phrase the null hypothesis and alternative hypothesis. The alternative hypothesis (Ha or H1) always states the objective of your research, the assumption you have, while the null hypothesis (H0) covers everything else. Here, you differentiate between a one-tailed test (either left or right), also referred to as a composite hypothesis, and a two-tailed test, also called a simple hypothesis. This can mean that the Ha focuses either on a critical region or on one single result.
-
Right-sided one-tailed test
Ha: There are more than 56% women working in the fashion industry. (Critical region)
Ha: µ > 56%
H0: There are less or equal to 56% women working in the fashion industry.
H0: µ < 56% -
Right-sided one-tailed test
Ha: There are less than 56% women working in the fashion industry. (Critical region)
Ha: µ < 56%
H0: There are more or equal to 56% women working in the fashion industry.
H0: µ > 56% -
Two-tailed test
Ha: There are 56% women working in the fashion industry. (Specific value)
Ha: µ = 56%
H0: There are more or less than 56% women working in the fashion industry.
H0: µ ≠ 56%
Data collection & significance level
The probably most important step is collecting your data. You can do this by using different sampling methods, conducting interviews or questionnaires, or any other methodology that suits your needs. However, you should always check for research biases, as they can highly influence the validity of your study in a negative sense or warp the results of your hypothesis testing.
The level of significance (α) is the probability of rejecting the null hypothesis when it is actually correct. Typically, the level of significance is set to 5%, but depending on your study, previous research or importance of the result, it can also be set to 1%, 10% or anything you deem suitable. Just in case this seems confusing: yes, you just define the level of significance. There is no calculation or procedure to determine it. If you are unsure of what error rate to use, you can ask your professor or look up what similar studies have used.
Selection and conduction of a test
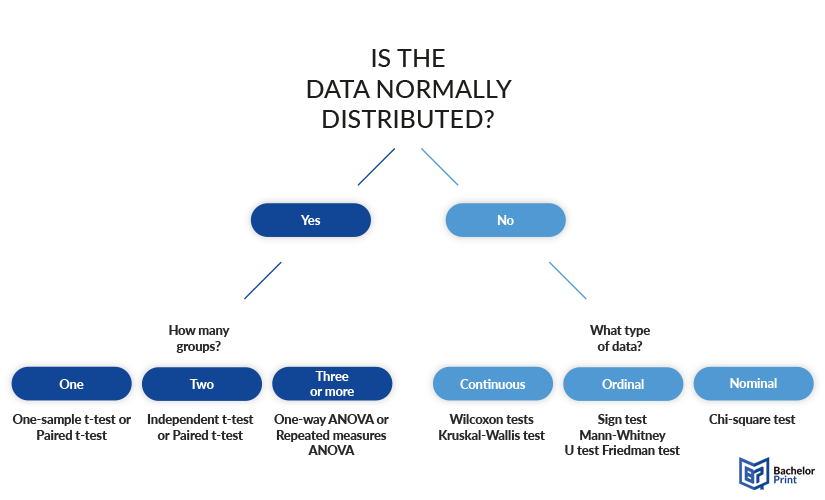
Select the fitting test for your type of data for your hypothesis test. The following chart will show you how to choose the right test. The first question you will have to ask yourself is whether your data is normally distributed or not. A normal distribution is always symmetric around its mean, which is the base for any statistical test.
If your dataset is normally distributed, you use parametric tests according to how many sample groups you used. These can include any type of t-tests and ANOVAs. If your dataset is not normally distributed, your decision depends on the types of variables present in your study, selecting either non-parametric tests or the Chi-square test, which is again parametric.
Decision
After calculating all relevant values, it is time to make a decision on one of the hypotheses. Usually, a researcher looks to reject the null hypothesis, since the alternative hypothesis states his opinion or assumption. In a t-test, for example, there are two ways to determine which hypothesis is less plausible.
- Reject H0 if the test statistic > critical value.
- Reject H0 if the p-value < the significance level (α).
Typically, you decide on one of the two ways, but both of them should give you the same result. If you want to know how to conduct each of the possible tests, take a look at our corresponding articles.
Presentation of the results
In a research paper, your thesis or a dissertation, the results of your hypothesis testing are presented in the results and discussion sections. The results section gives a brief summary of your findings, stating objectively what your research found. In the discussion, on the other hand, you will comment on whether the results supported or rejected your primary assumption and what this tells you about your research topic.
When writing your thesis, keep in mind that hypothesis testing only defines the most plausible hypothesis and never proofs one of them for good. These tests are based on a sample, which always has a possibility of varying from the actual population. Moreover, if you reject the null hypothesis, you will not state that directly but rather emphasize the findings that support your alternative hypothesis. However, if, against your assumptions, you had to reject your Ha, it might be interesting if you discuss this more closely and describe the reasons for the situation.
Hypothesis Testing: Example
Peppermint Essential Oil (t-test)
Chamomile, lavender, and peppermint are some of the most popular essential oils available today. Having heard about their ability to reduce anxiety, you may want to prove whether this essential oil does indeed have some healing powers.
In this case, the hypothesis is likely to go as below:
I. The null hypothesis—As an essential oil, peppermint doesn’t assist in reducing anxiety pangs
The alternative hypothesis—Peppermint is capable of reducing anxiety pangs
II. Level of significance—Place the significance level at 0.25 (this will provide you with a better opportunity to prove the alternative hypothesis).
III. P-value—It’s calculated as 0.05
IV. Conclusion—Once one of the groups is proven using a placebo and the other with peppermint oil, you will need to differentiate the two according to the self-reported anxiety levels. Using your calculations, any difference that exists between the two test groups will be statistically important when it has a 0.05 p-value. This is well-below the pre-defined 0.25 alpha level. The conclusion will, therefore, note that the results of your examination support the alternative hypothesis.
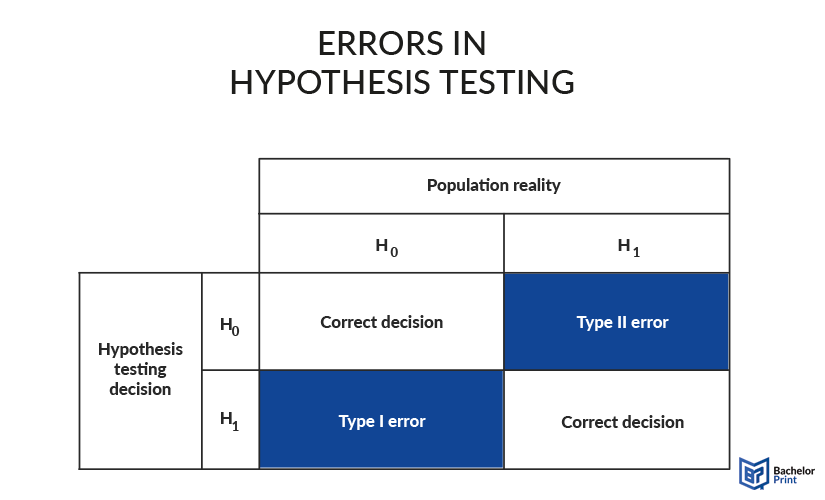
Type I and type II errors
Type I and type II errors are possible mistakes in the decision for one hypothesis. They are also sometimes referred to as α and β mistake. Both of them originate from assuming the wrong hypothesis as right. The first type of error occurs when the null hypothesis is falsely rejected. The second type of error happens when H0 is falsely accepted. Both of them can lead to a wrong conclusion on the population standard.
Applications of hypothesis testing
Hypothesis testing is, of course, used in academic writing and scientific papers. However, there are also many applications outside academic science. Fields like for example economy, healthcare and manufacturing use hypothesis testing to gain valuable insights on their target groups or market development.
Examples
-
Healthcare: In medical research, this procedure is often used to determine whether a new drug is effective or not. Then, the data collected from the sample and control groups are used to conduct a hypothesis test, which hopefully results in the medicine being effective.
– H0: The medication has no effect.
– H1: The medication has the desired effect. -
Manufacturing: In factories and other producing companies, it is used to conduct quality-checks, determining if a process meets the required standards or not.
– H0: The final product weighs more or less than 450g.
– H1: The final product weighs 450g. -
Market research: In market research, hypothesis testing is used to determine the success of marketing strategies or consumer behavior.
– H0: The new marketing campaign increases sales less than 10% or not at all.
– H1: The new marketing campaign increases sales at least 10%. -
Economy: In economics and finance, it is used to test theories and the impact of new measures or policies on economic growth, as well as investment decisions.
– H0: The new policy has no impact on economic growth.
– H1: The new policy has a positive or negative impact on economic growth. -
Environmental science: In environmental sciences, hypothesis testing is used to calculate the impact of different factors on our natural environment, whether it is pollution, emission of greenhouse gasses or surface sealing.
– H0: Environmental pollution has no impact on plant growth and health.
– H1: Environmental pollution has a great impact on plant growth and health. -
Social science: Social sciences like sociology, psychology, or political sciences use this methodology to investigate hypotheses on social behavior and other complex components of human nature.
– H0: Pets don’t have any impact on mental health.
– H1: Pets have a positive impact on mental health.
Hypothesis Testing – FAQs
Before it comes to printing & binding your dissertation, you need to do your research, which often includes hypothesis tests. There is no variation in the manner in which hypothesis tests are performed or written. In hypothesis testing, the researcher should start by stating the hypothesis that they intend to examine. From here, they will need to formulate a plan on how to conduct the analysis, and then study their sample data. Sample data analysis is then followed by the acceptance or rejection of the null hypothesis established earlier.
An examinable hypothesis is never a simple statement. The researcher has to come up with an intricate statement capable of providing a flawless overview of the scientific experiment at hand. It should also state the intentions of the experiment and the outcomes likely to be achieved. During hypothesis testing, you will need to consider the following:
I. Start by stating the problem you would like to solve.
II. If possible, ensure the hypothesis you craft appears in the form of an if-then statement.
III. Outline all your variables.
Hypothesis testing generally has five steps:
- formulating of the hypotheses
- data collection and determination of the level of significance
- selection of the suitable test plus its conduction
- decision to accept or reject the null hypothesis
- presentation of the results
For hypothesis testing, you usually use one of these statistical methods: a t-test, an ANOVA or a Chi-square test.
